EQUATION OF TIME
Astronomy
The equation of time is an astronomical concept that quantifies the difference between true solar time and mean solar time throughout the year. This discrepancy arises from the irregularity of the Earth’s orbit around the sun combined with the tilt of its axis. The concept is crucial for understanding why solar noon (when the sun reaches its highest point in the sky, the zenith) does not correspond precisely to the time displayed on our watches and clocks.
Influenced by these two factors, the duration of an astronomical day (true solar day) is not constant and fluctuates throughout the year based on the Earth’s position around the sun. Conversely, our calendars and time-measuring systems use days of constant average duration (24 hours) throughout the year (mean solar day). The equation of time expresses the difference between these two values.
Factors
- The Earth’s Elliptical Orbit
The Earth follows an elliptical orbit around the sun. It moves faster when closer to the sun (perihelion, around 3rd January) and slower when farther away (aphelion, around 4th July) (Kepler’s Law). As a result, the interval between two successive solar zeniths at a given point lengthens when the Earth is farther from the sun and shortens when it is nearer. - The Tilt of the Earth’s Axis
The Earth’s axis is tilted approximately 23.5° relative to its orbital plane. This tilt causes seasonal variations in the sun’s angle of illumination, affecting its apparent position in the sky.
The equation of time results from the combination of these two effects, represented as a function that varies throughout the year. It can be expressed mathematically, showing the deviation (in minutes) between mean solar time and true solar time.
Value of the Equation of Time
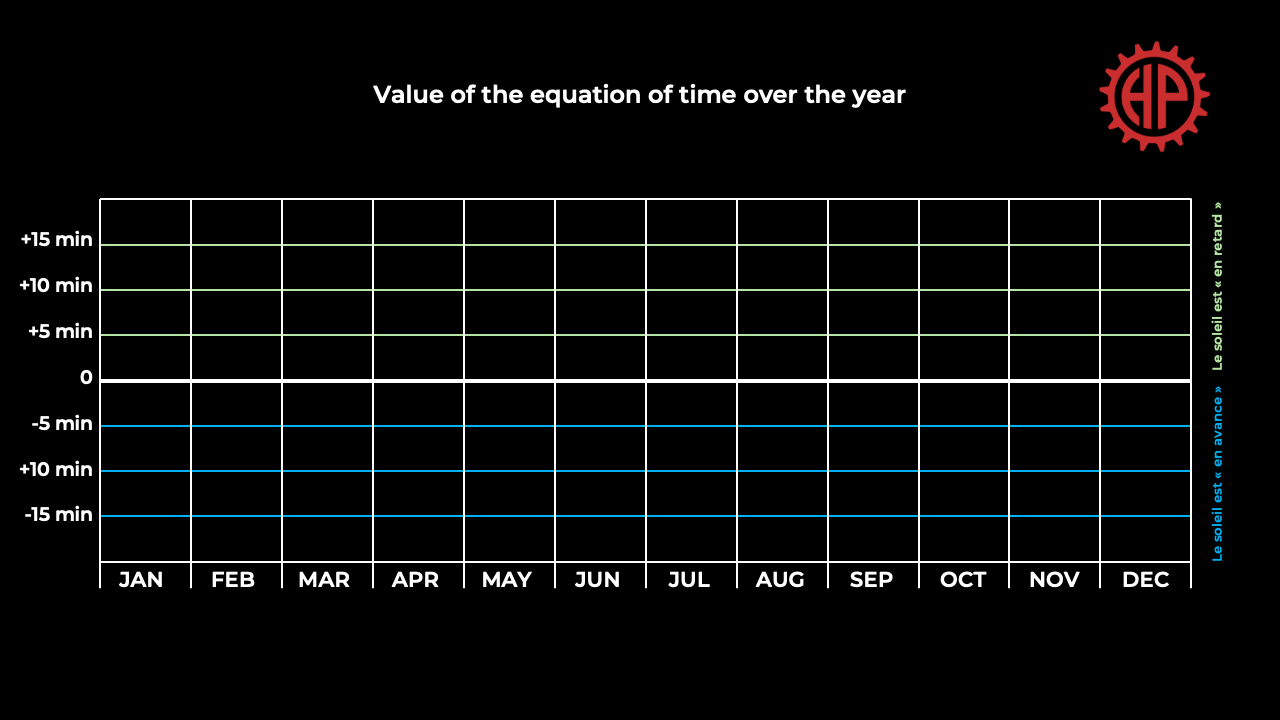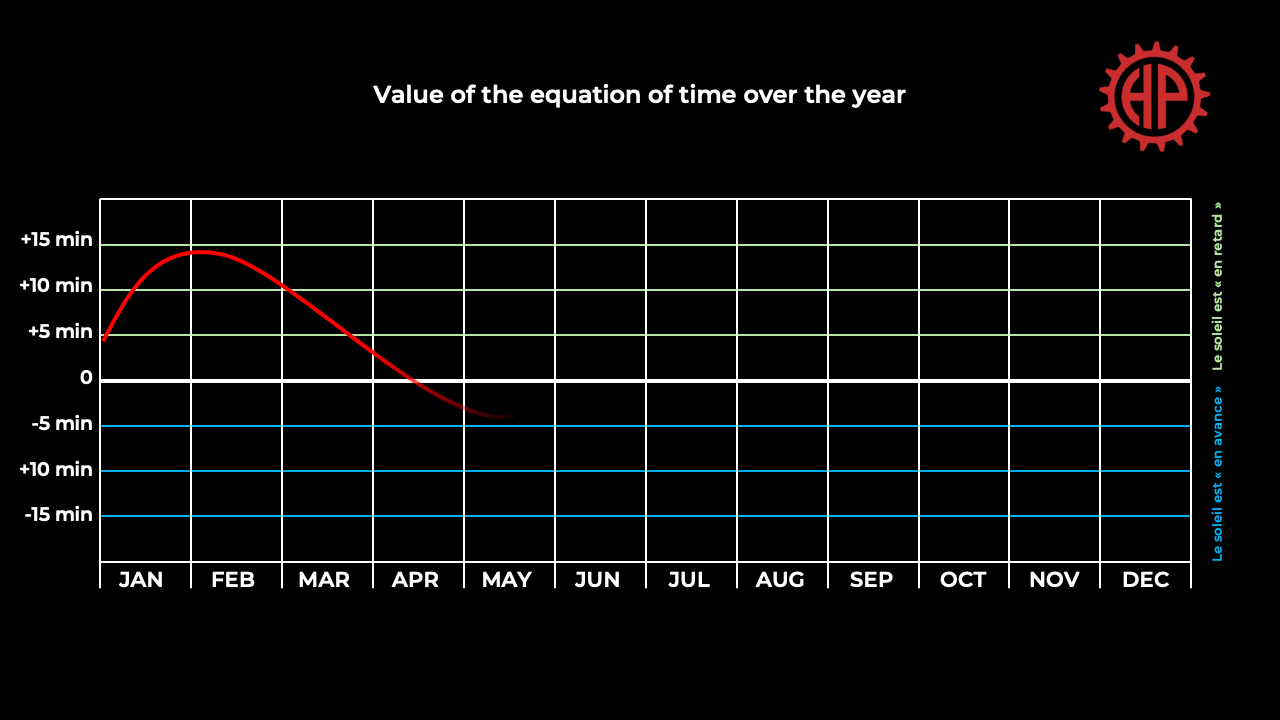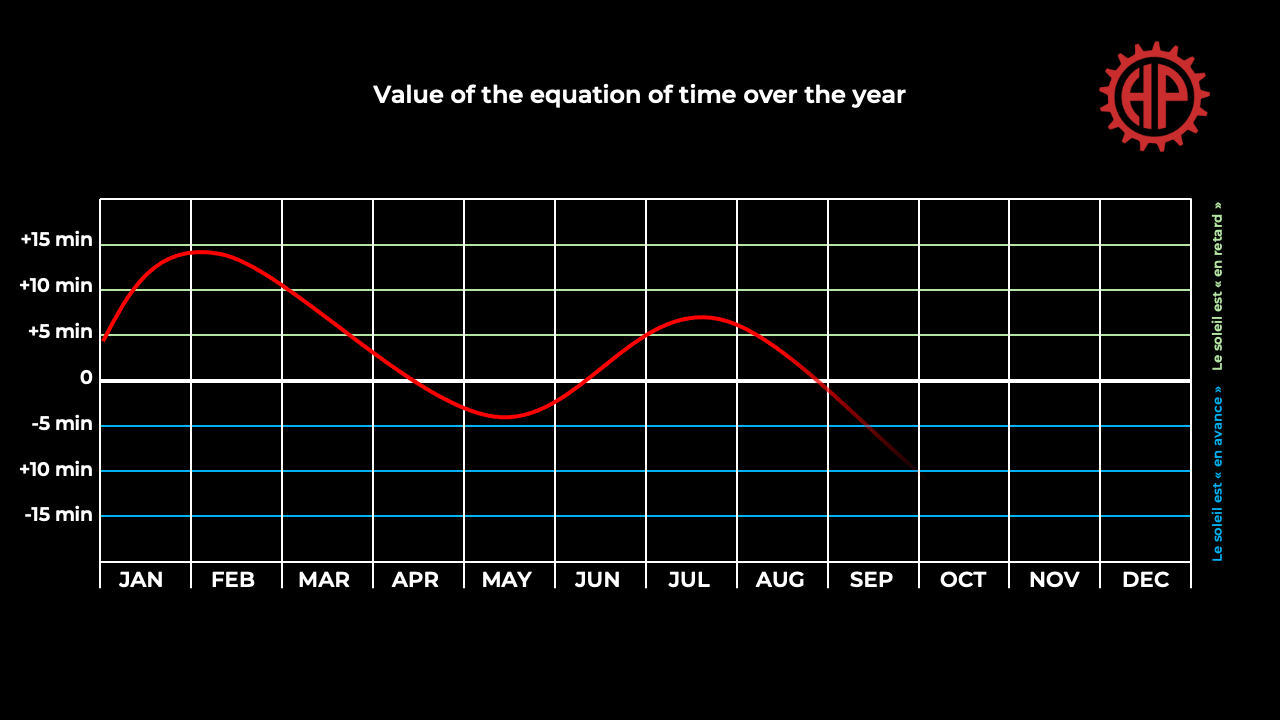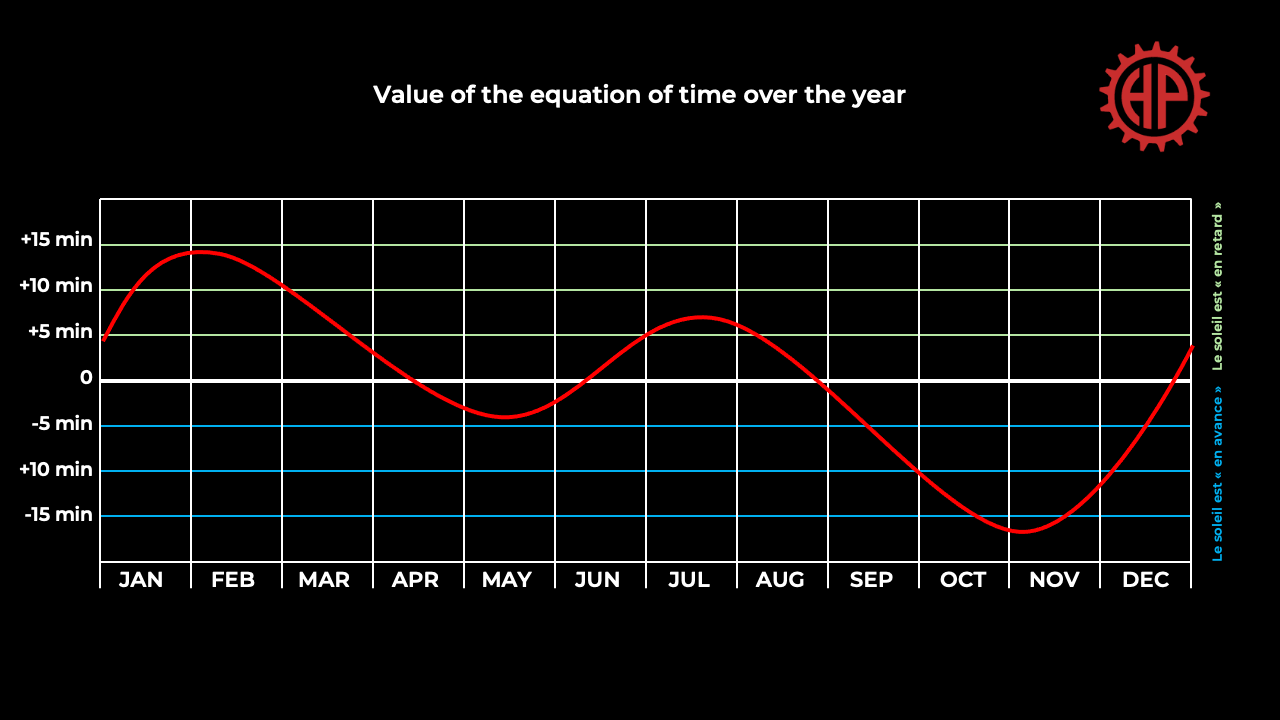
The amplitude of the equation of time reaches approximately 16 minutes. This difference varies throughout the year, with maximum values occurring in spring and autumn, and minima around the winter and summer solstices. For instance, in February, the equation of time may be +14 minutes (the sun appears “ahead” of mean solar time), whereas in November, it can reach around -16 minutes (the sun appears “behind” mean solar time).
Practical Applications
The equation of time is crucial for several applications, including:
- Astronomical Navigation: When GPS systems fail, sailors use true solar time to determine their geographic position. The equation of time is essential for correcting the time displayed on a watch.
- Sundials: Sundials must account for the equation of time to provide an accurate reading of true solar time.
- Astronomy and Solar Observations: The equation of time allows astronomers to adjust the time displayed by a standard watch or chronometer to obtain accurate solar time for their observations.
The equation of time is an astronomical phenomenon resulting from the Earth’s elliptical orbit and the tilt of its axis. These factors cause variations in true solar time relative to mean solar time. While the term “equation of time” might suggest a complex mathematical problem, it is, in fact, a periodic function with practical uses in fields such as navigation, astronomy, and time measurement.
In practical terms, an equation of time mechanism is relatively simple and does not require many components. However, its drive system deserves special attention, and its components must be designed and manufactured with high precision to ensure the accuracy of the displayed information.
The astronomical section of this chapter has shown us that the annual curve of the equation of time resembles the following:
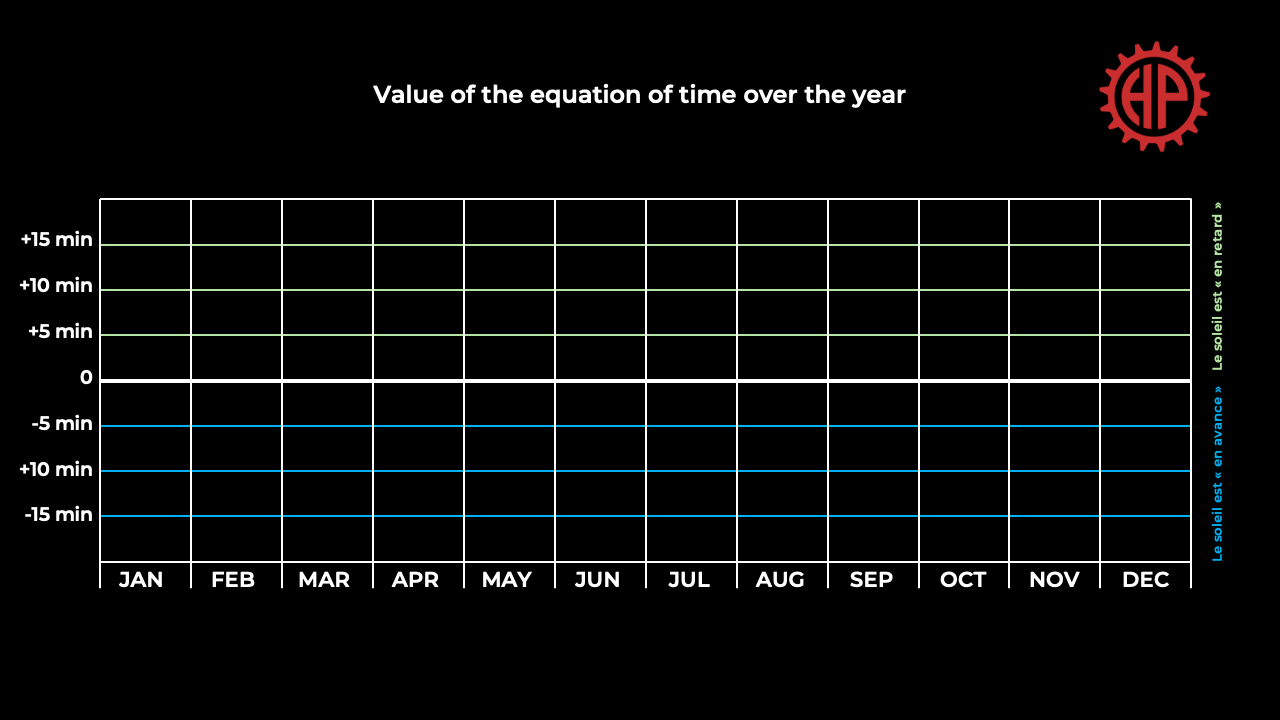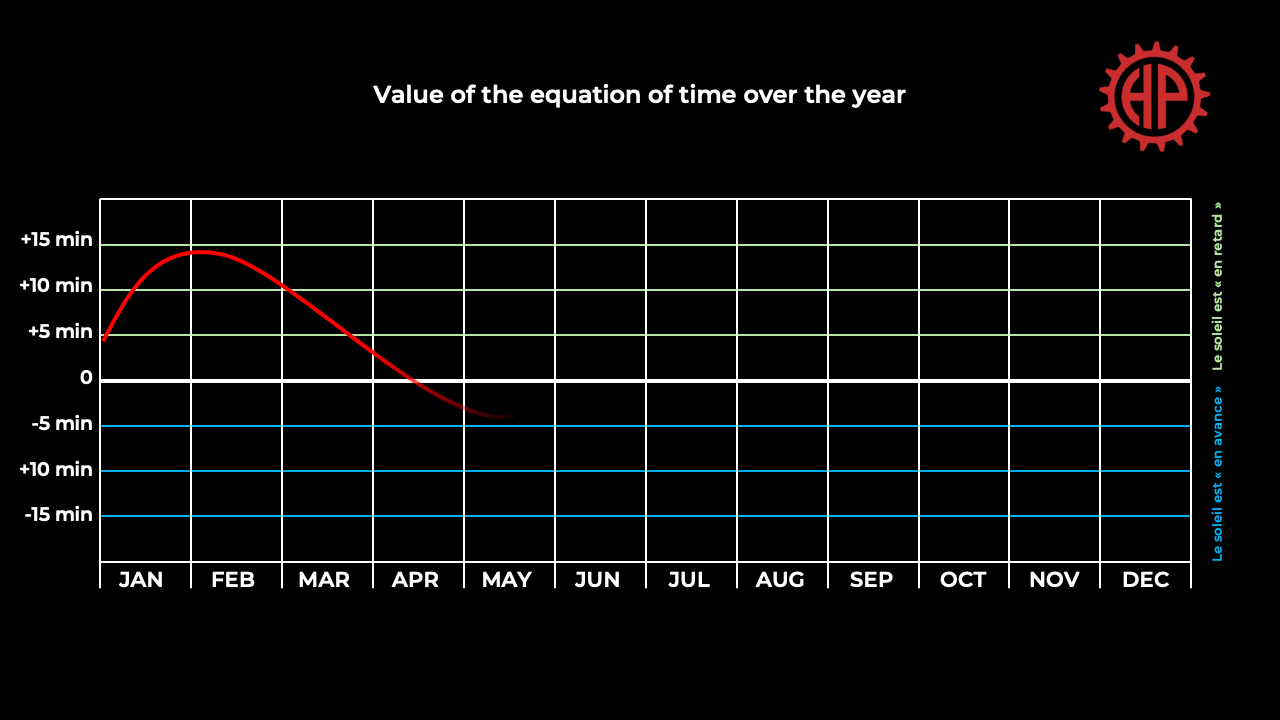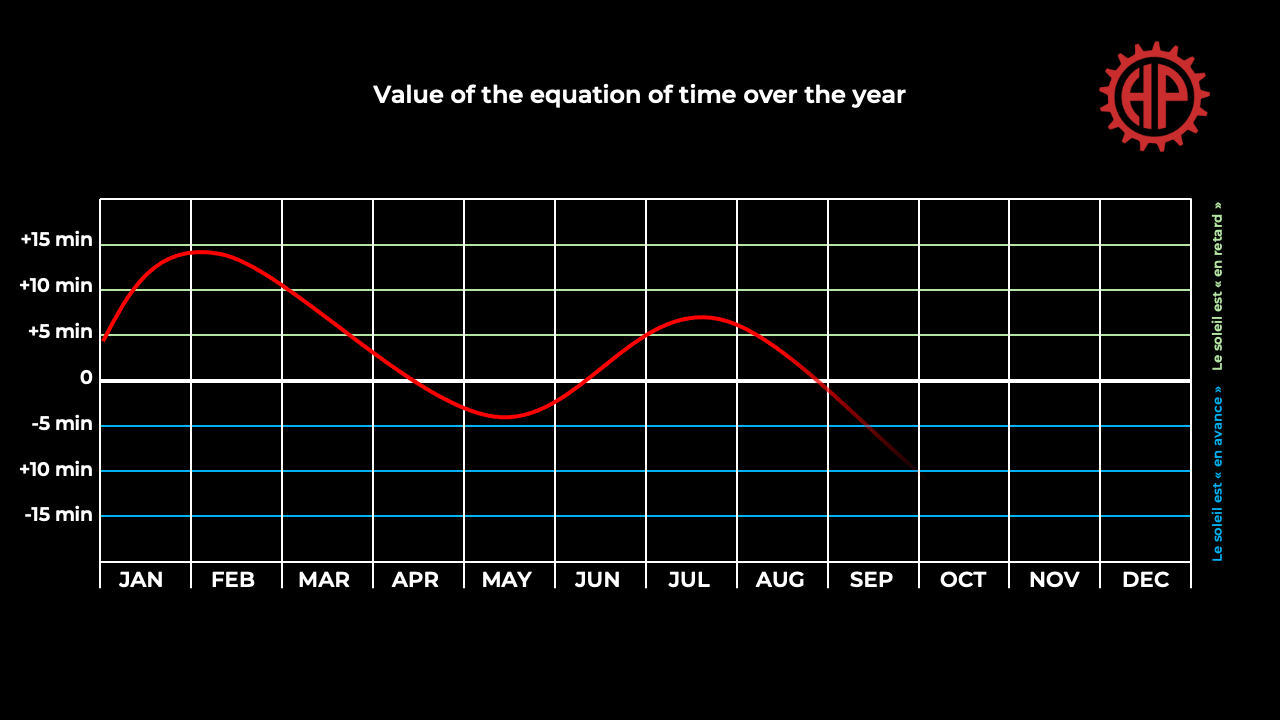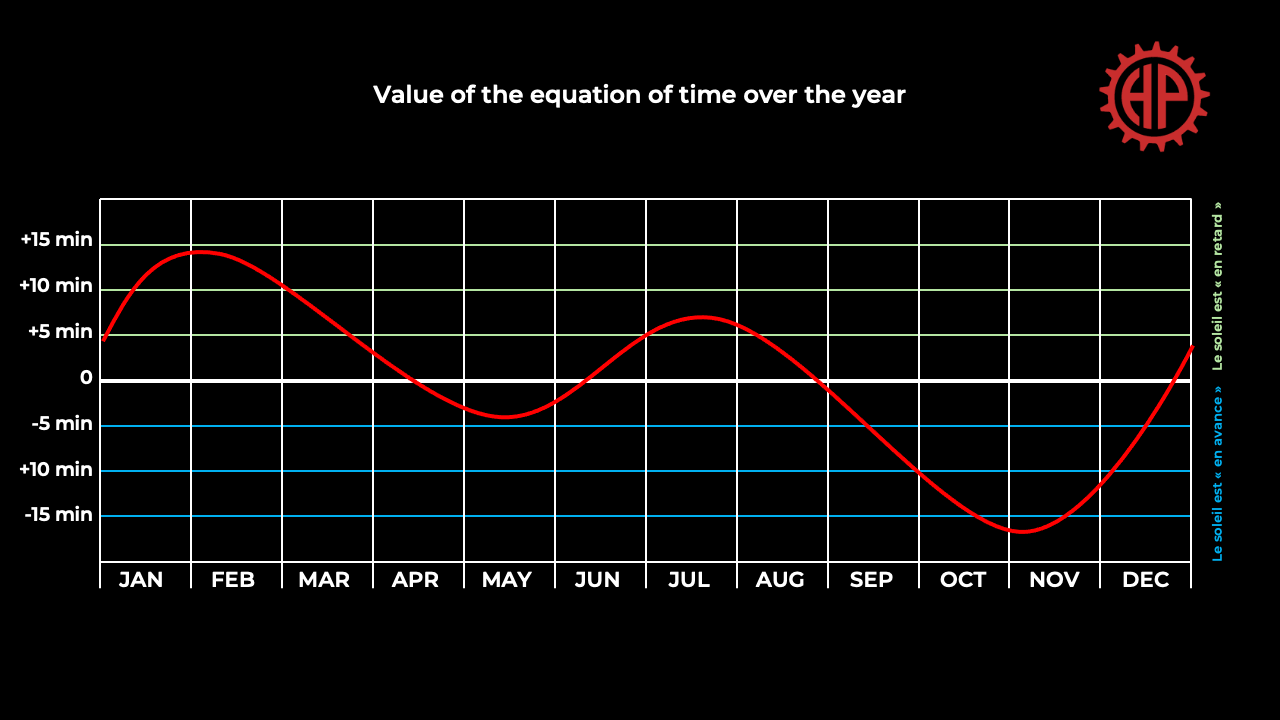
Design of the Equation of Time Cam
To mechanically translate this information, it is necessary to convert this curve into a circular expression to design a cam that will control the equation of time indicator. The circular projection of this curve corresponds to the following model:
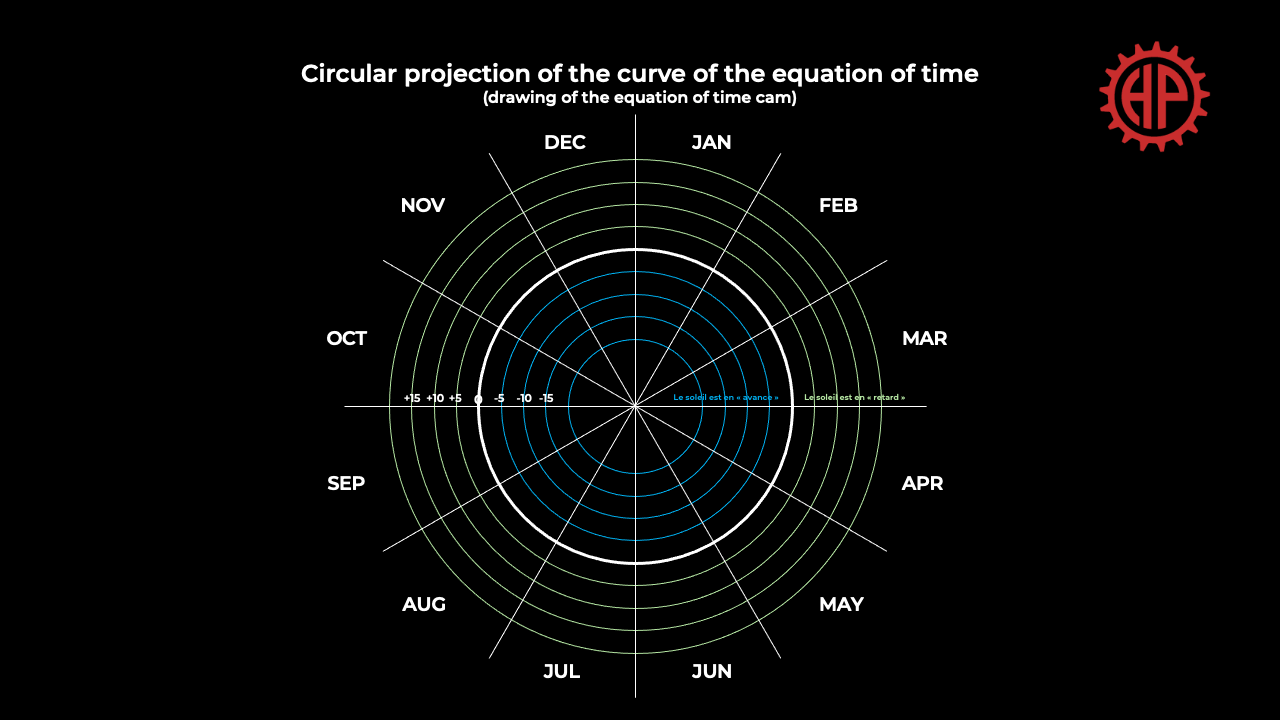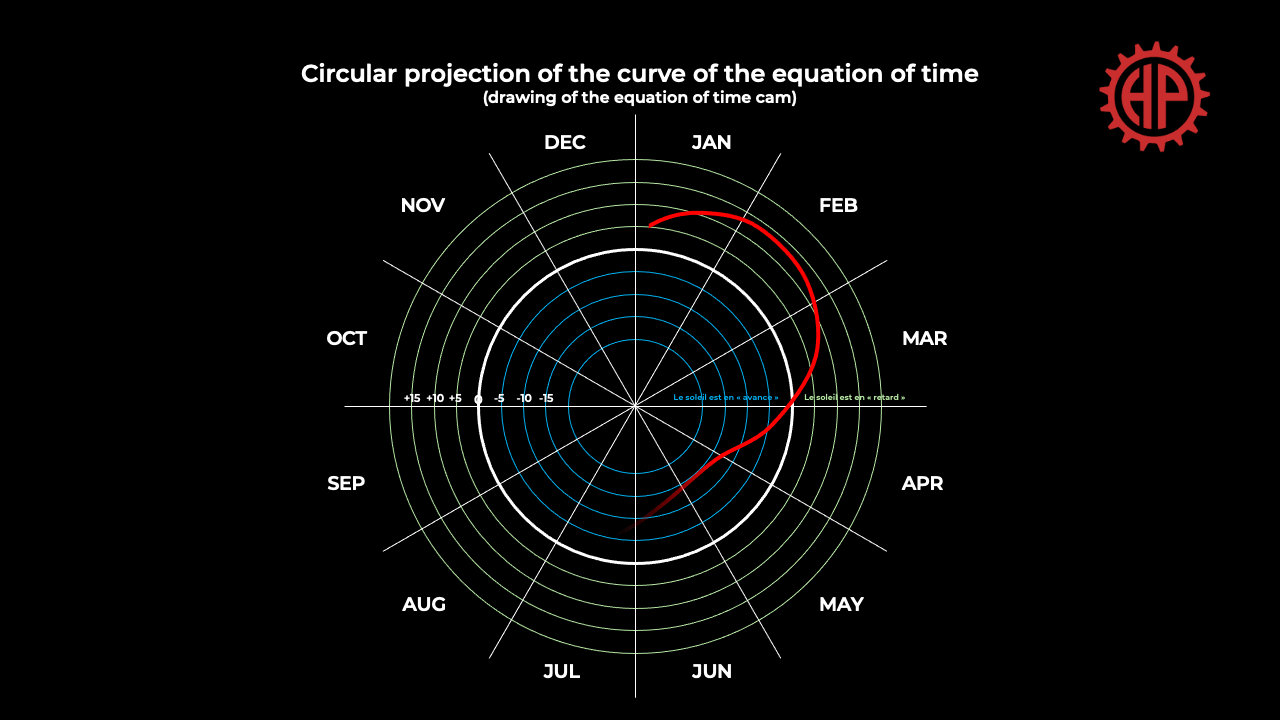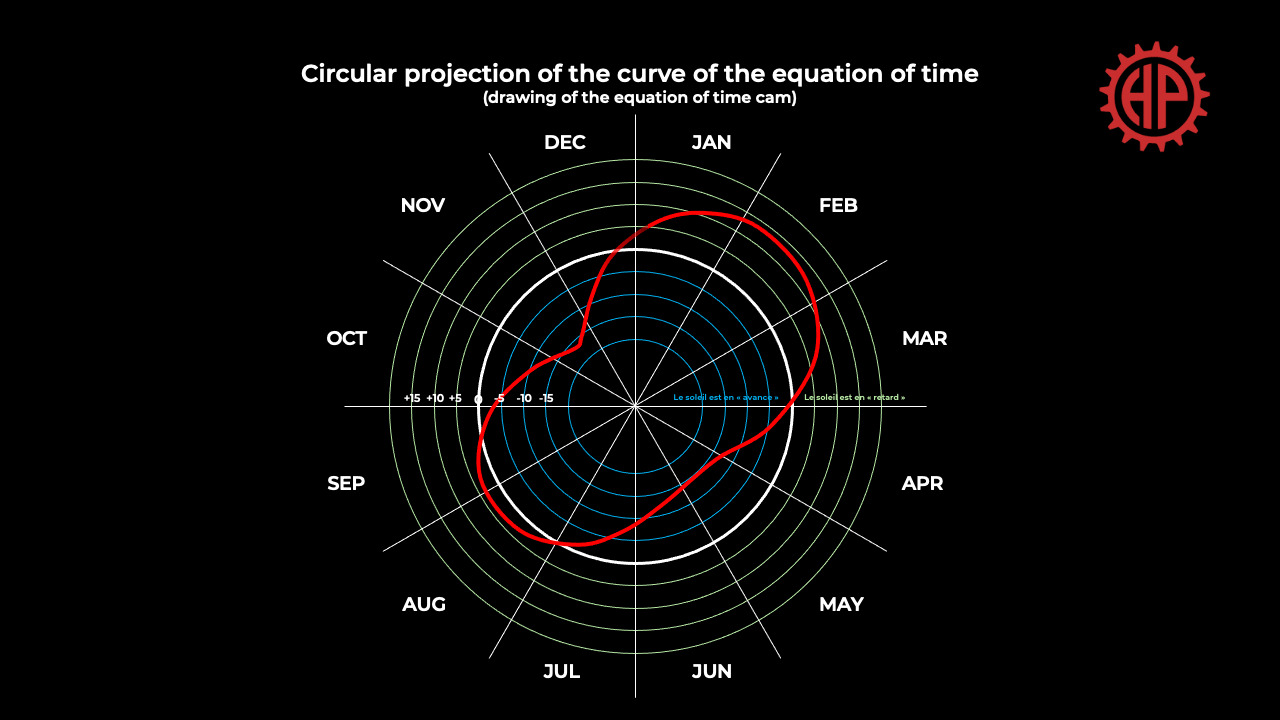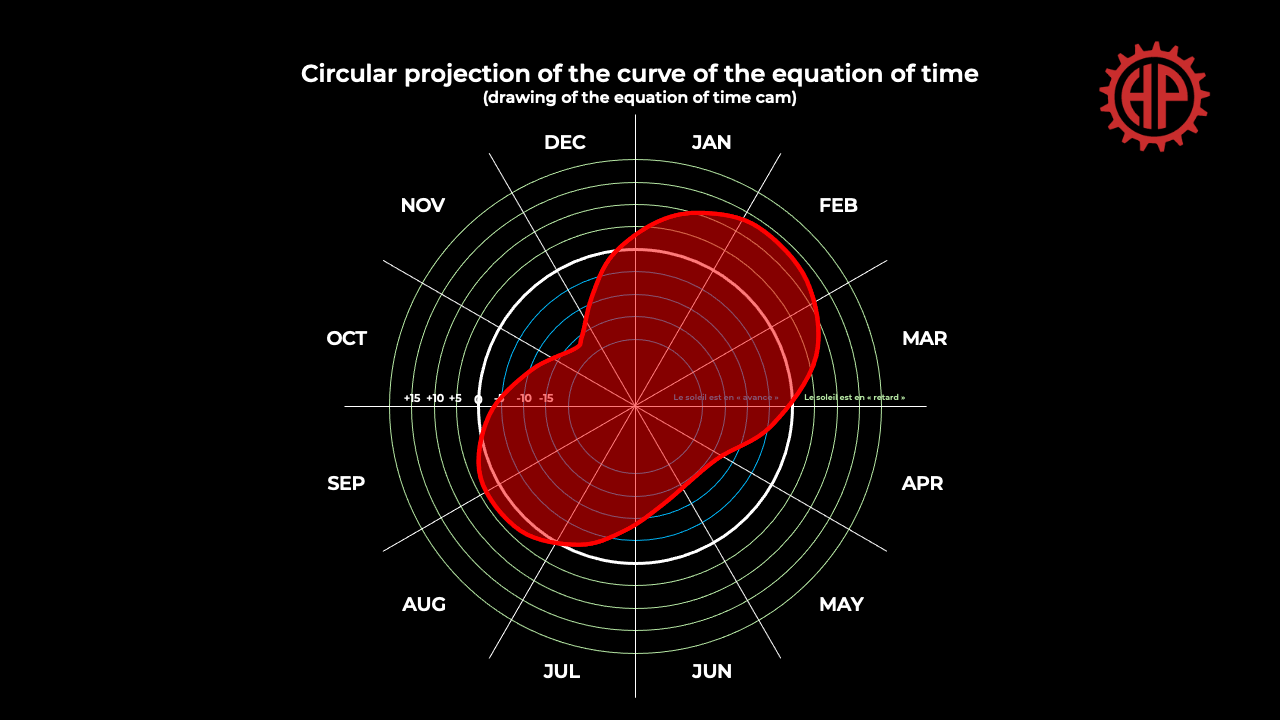
This curve can then be directly used to determine the exact shape and contour of the equation of time cam. The cam, once designed, acts as the mechanical program for displaying this function.
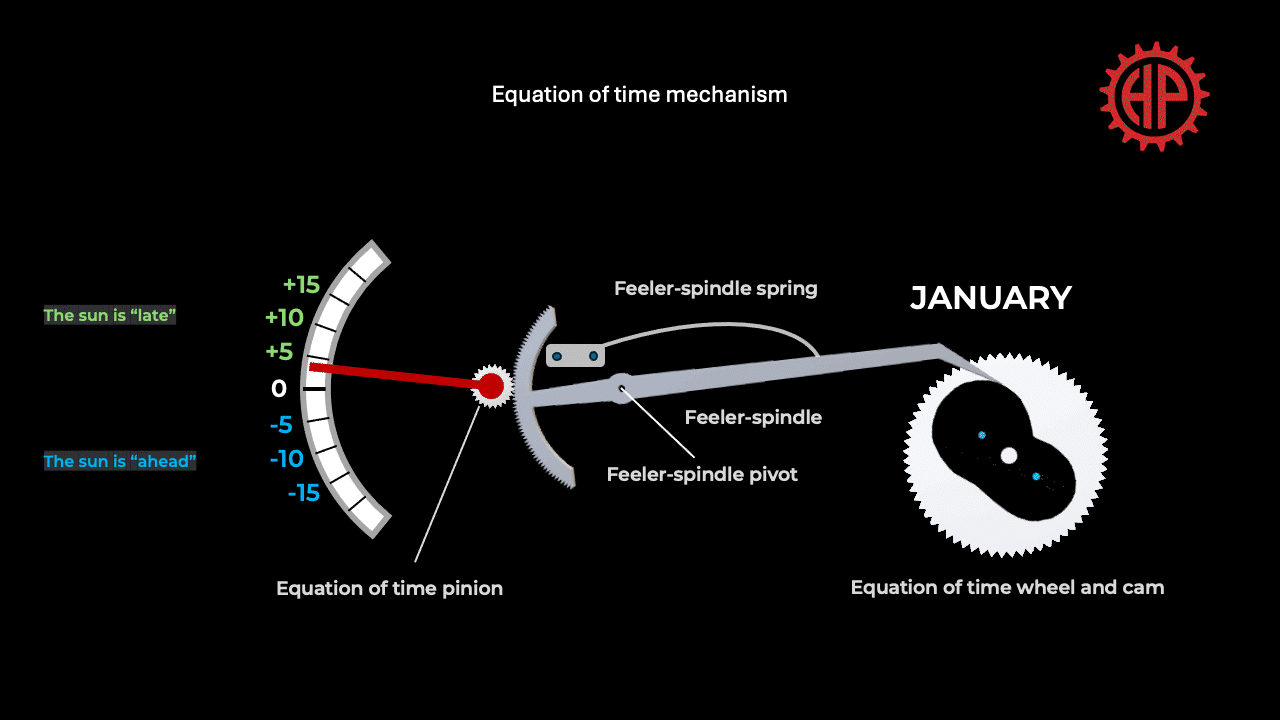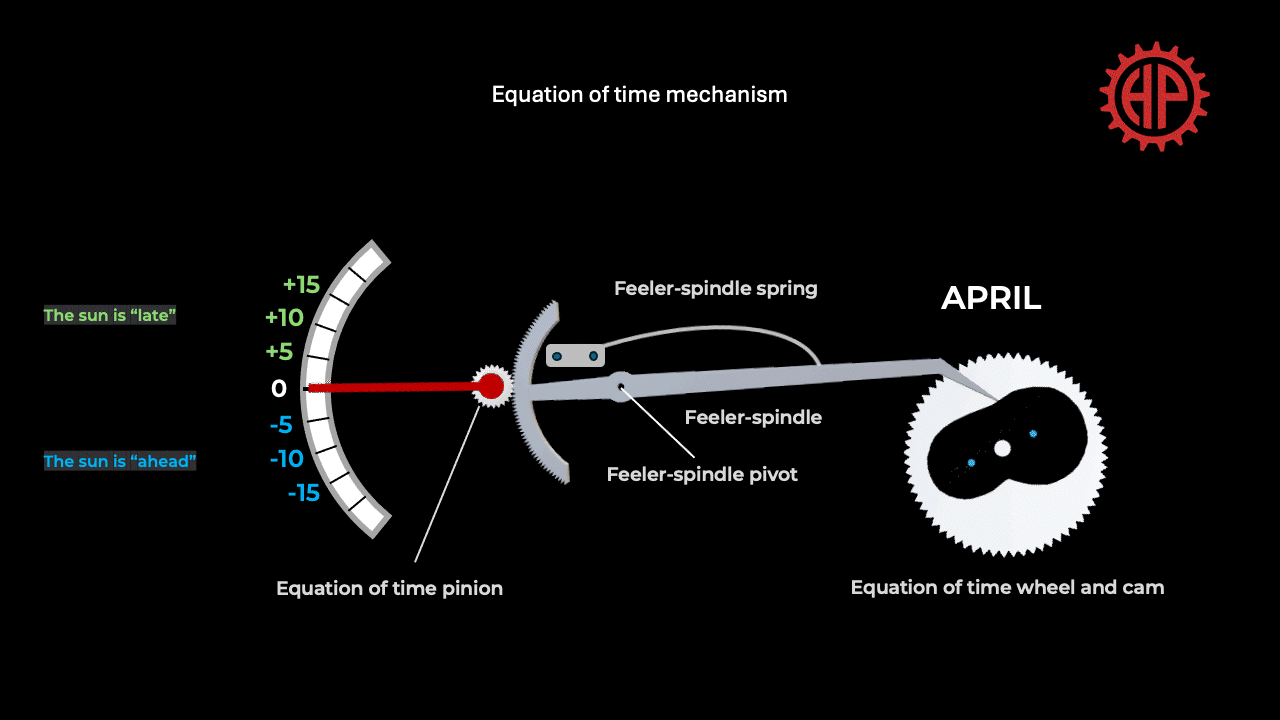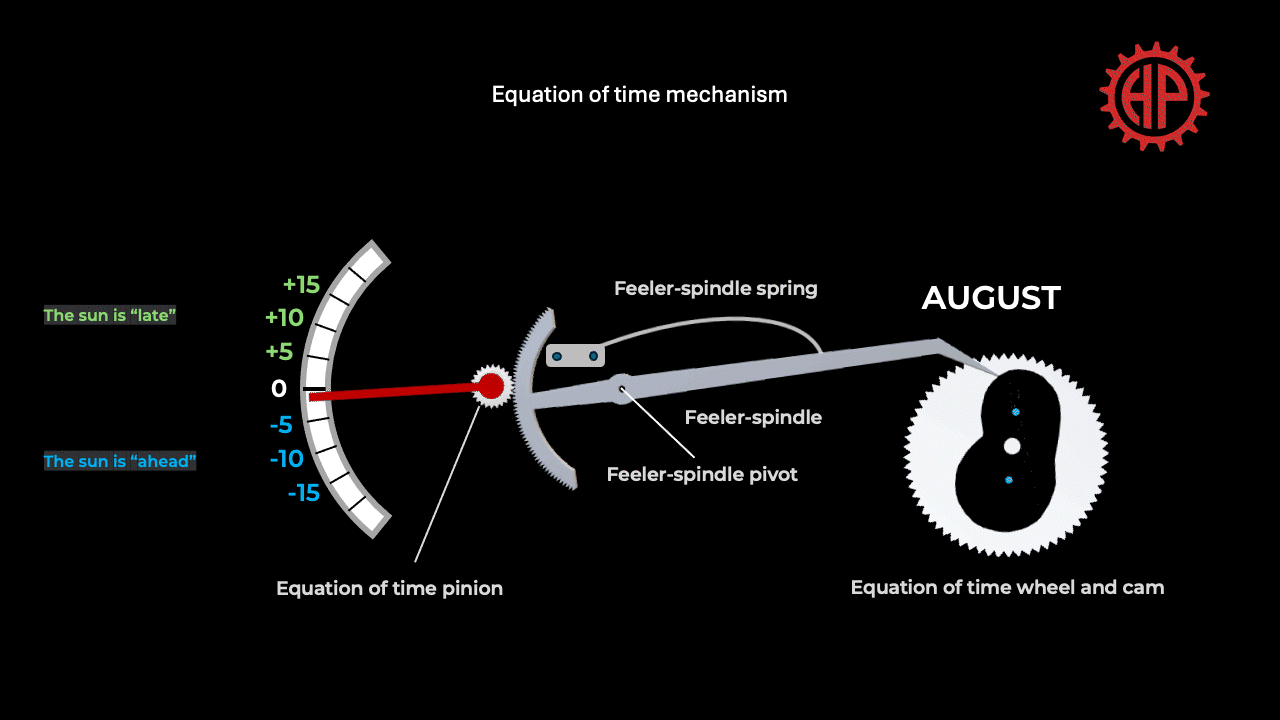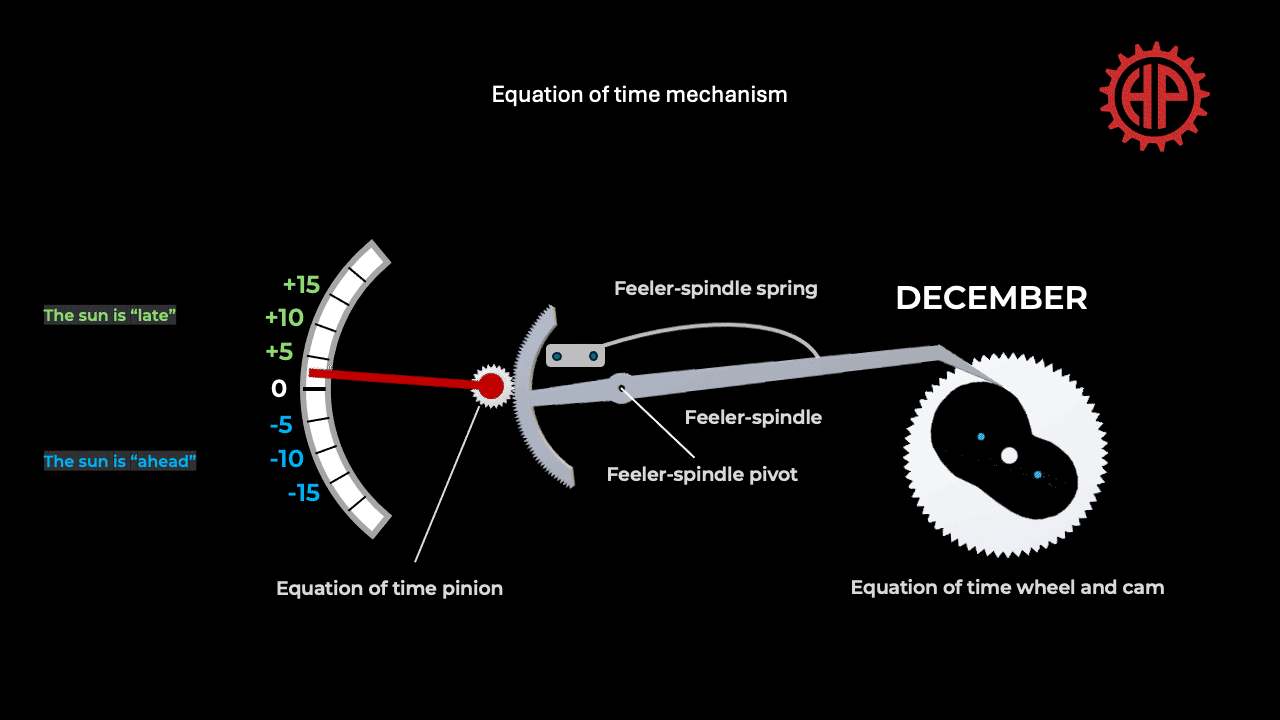
Operating Principle
The equation of time cam is fixed to the equation of time wheel, which is generally driven by an intermediate gear.
The equation of time wheel must complete one revolution in an astronomical year, which is 365.25 days. It is therefore incorrect to drive an equation of time mechanism using a perpetual calendar based on the Gregorian calendar, which divides the leap year cycle into three years of 365 days and one year of 366 days. Using a perpetual calendar to drive the equation of time mechanism introduces an error of 0.25 days per year, accumulating to 0.75 days over three years. This discrepancy can cause a relatively significant reading error. To avoid this, it is preferable to use an annual calendar or, better still, to specifically calculate a gear train to ensure the correct rotational speed of the equation of time wheel (1 rotation/365.25 days).
A feeler-spindle, pressed against the contour of the cam by a spring, is located at the end of a large pivoting lever.
As the cam rotates, it moves the feeler, which pivots in one direction or the other, following the cam’s contour.
The opposite end of the feeler-spindle, located on the far side of its pivot point, terminates in a toothed sector (a rack). This rack meshes with the equation of time pinion, which carries the equation of time hand. The angular value displayed is determined by the gear ratio between the feeler’s toothed sector and the equation of time pinion.
This description outlines the precise mechanical operation and the careful engineering required to implement the equation of time in a timepiece, ensuring both accuracy and elegance in its execution.